This post is a followup to my earlier example of the syntonic comma in musical tuning. In my original post, the goal was to arrive at the “comma sibling” of our starting note, C, by traversing a series of four ascending fifths followed by a descending major third. We saw how this sequence, when tuned using pure 3/2 fifths and 5/4 major thirds, leads us to a C’ that’s sharp of an octave above our starting note. In this post we’ll explore the same tuning discrepancy, but instead of considering two versions of C we’ll consider two versions of the sixth scale degree, A, differing by the same amount as our C’s.
Here’s how our two versions of the sixth degree arise:
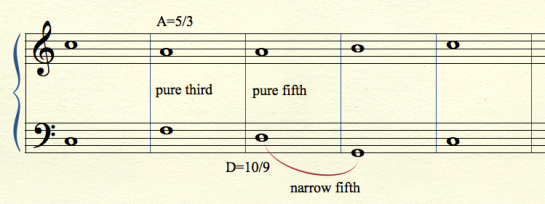
Version 1: If we start at F, tuned to 4/3, or a pure perfect fifth below C, we can traverse a sequence of four ascending perfect fifths (F->C->G->D->A). We arrive at a pitch with the ratio 27/16 (that’s 4/3 x 3/2 x 3/2 x 3/2 x 3/2) which is the sharper of the two A’s.
Version 2: If we start at F, we can ascend by a pure major third. We arrive at a pitch with the ratio 5/3 (that’s 4/3 x 5/4), which is the flatter of the two A’s.
Now we’ll look at how those two A’s function in the context of a very simple melody line above a bass.
Example 1:
Here we introduce the 5/3 A in measure two and of course it makes a pure major third above the F in the bass. However, it creates a narrow fifth with the 9/8 D in the bass in measure three. (We want that D to be tuned at 9/8 so that it will make a pure perfect fifth with G at 3/2.) Listen to how the interval in measure two sounds smooth and calm while the interval in measure three wobbles.[audio http://rudiseitz1.files.wordpress.com/2013/12/syntonic11.mp3]
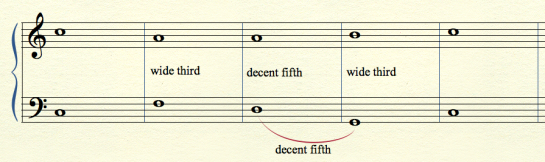
Example 2:
Here we use the sharper 27/16 A. In measure two it creates a wide third with F in the bass (listen to how there’s a bit more commotion or discord here than before) but in measure three, it creates a pure fifth against D.
[audio http://rudiseitz1.files.wordpress.com/2013/12/syntonic21.mp3]
Example 3:
To have pure intervals in both measures two and three, we could try using the two A’s in sequence. We’ll employ the 5/3 A over the F and then switch to the sharper 27/16 A over the D, so each A will be perfectly matched to the bass note. Indeed this will give us the pure intervals we want, but the slight rise in pitch in the top line between measures two and three could be disconcerting; listen and see what you think.
[audio http://rudiseitz1.files.wordpress.com/2013/12/syntonic31.mp3]
Example 4:
Another possibility is to lower the tuning of D in the bass in measure three so it makes a pure perfect fifth with 5/3 A, which we’ll use throughout. Unfortunately, lowering the D means that it will no longer make a pure perfect fifth with the following G in the bass. This might not be so bad. The D-G mistuning is hard to hear because it occurs melodically (not as a simultaneous interval), and because it’s in such a low register. One could make a case for this being the cleanest option considered so far, though that slightly flattened D could cause trouble elsewhere if we continued to use it in an extended composition.
[audio http://rudiseitz1.files.wordpress.com/2013/12/syntonic41.mp3]
Example 5:
Of course, equal temperament eliminates the distinction between our two A, and gives us wide major thirds along with fifths that are close to pure. In this equal-tempered clip you’ll notice there’s a bit of wobble in measure 2 as well as, for the first time, in measure 4.
[audio http://rudiseitz1.files.wordpress.com/2013/12/syntonic51.mp3]
Example 6…
Are there more possibilities to explore? Yes. We should look at how this passage works in a meantone temperament, which eliminates the syntonic comma without imposing the total uniformity of equal temperament. But that will have to wait till another post. ■