There’s an idea floating around that the Lydian Dominant scale (1, 2, 3, #4, 5, 6, b7), defined in the context of twelve-tone equal temperament, is somehow special because it emulates a harmonic series. Sometimes the Lydian Dominant scale is called the “acoustic scale” or the “overtone scale.” Those names strike me as misleading. The idea is that if we look at the harmonic series of C2, for example, we find something roughly similar to these notes from the piano: C2, C3, G3, C4, E4, G4, Bb4, C5, D5, E5, F#5, G5, A5. Arranging the distinct notes from that sequence into an octave starting at C gives us C, D, E, F#, G, A, Bb. This scale might have some wonderful properties, but can those properties really be explained by the scale’s resemblance to the harmonic series?
In fact it’s a stretch to say this scale is “based on the harmonic series” at all because the tuning discrepancies are so vast. The members of the harmonic series do not match the piano’s C, D, E, F#, G, A, Bb – you can’t play any portion of the harmonic series accurately on a conventionally tuned piano. The piano’s G and D are reasonably close to the third and ninth partials of C; E is far off from the fifth partial; Bb and F# are still further off from the seventh and eleventh partials; and A is even further off from the thirteenth partial (which is actually closer to Ab).
Alright then, even if we acknowledge the discrepancy between a true harmonic series and the notes we can access on the piano, might there still be something useful or interesting about approximating the harmonic series through our 1, 2, 3, #4, 5, 6, b7 scale – might the ear tolerate the discrepancies and still hear some special cohesion in those notes on the piano because of the way they mimic, if not exactly match the harmonic series?
One way to get at this question is to flip it around. We know that the ear does perceive a special cohesion in a set of simultaneous pitches arranged in an exact harmonic series with decaying amplitudes – we generally perceive this phenomenon as a single note. So what would happen if we were to take the harmonic series of C and adjust its pitches so that they aligned with the piano’s C, D, E, F#, G, A, Bb – would these “tempered harmonics” still seem to fuse into a single note?
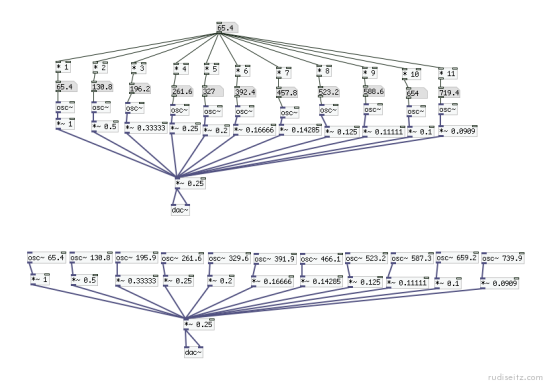
Here is a sound composed of the first eleven partials of C2 (65.4 hz), where the amplitudes decay as 1/n where n is the number of the partial:
[audio http://rudiseitz1.files.wordpress.com/2014/01/harmonicovertones.mp3]
And here is a sound built from the equal-tempered pitches of the Lydian Dominant scale, arranged as an approximate harmonic series – C2, C3, G3, C4, E4, G4, Bb4, C5, D5, E5, F#5 – with the same decay pattern as before. In other words, this how our C2 sounds if all its partials are tuned to be playable on a piano:
[audio http://rudiseitz1.files.wordpress.com/2014/01/temperedovertones.mp3]
If your perceptions are at all like mine, you’ll hear the first example as a clearly defined steady pitch. The second example is less steady (there’s some wobbling or beating) and if you listen closely you can begin picking out individual components of the sound which don’t quite fuse into a seamless whole – the texture is “messy” and/or “chaotic.”
What can we conclude from this? I think it’s safe to say that the special perceptual properties of the harmonic series start breaking down when the harmonics are mistuned. If the mistuning is carried so far as to bring the harmonics in line with pitches of twelve-tone equal temperament, the difference in sound is quite drastic: the components cease to fuse. There may be great creative value in taking inspiration from the harmonic series and trying to build structures in twelve-tone equal temperament that mimic it, but if the result sounds good, we shouldn’t be too quick to assume the goodness comes directly from some resemblance to the harmonic series; any such resemblance is limited by a very significant difference in tuning.
PureData patches used to generate the sounds clips:
■